Electricity: 10th CBSE Science Physics

Question: Show how you would connect three resistors, each of resistance 6 Ω , so that the combination has a resistance of (i) 9 Ω , (it) 4 Ω .

Answer: (i) In order to get a resistance of 9 Ω from three resistors, each of resistance 6 Ω, we connect two resistors in parallel and this parallel combination (of resistance 6 Ω, 6 Ω) in series with the resistor as shown below:

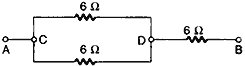
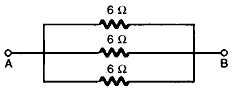
(ii) We cannot obtain a resistance of 4 Ω by any combination of three 6 Ω resistors. But we can obtain a resistance of 2 Ω, by connecting all of them in parallel as shown below:

Question: Several electric bulbs designed to be used on a 220 V electric supply line, are rated 10 W. How many lamps can be connected in parallel with each other across the two wires of 220 V line if the maximum allowable current is 5 A?

Answer:

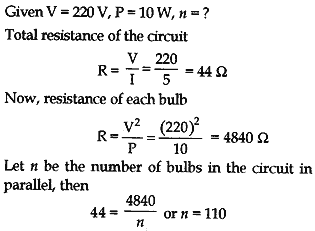
Question: A hot plate of an electric oven connected to 220 V line has two resistance coils A and B, each of 24 Ω resistance, which may be used separately, in series, or in parallel. What are the currents in the three cases?

Answer: Given  V = 220 V, R = 24 Ω, I = ?

(i) When each of the coils A or B is connected separately, current through each coil, i.e.,
I = V/R = 220/24 = 9.17 A

(ii) When coils A and B are connected in series, equivalent resistance in the circuit,
R_s = R + R = 24 + 24 = 48 Ω
Therefore, current through the series combination, i.e.,
I = V/R = 220/48 = 4.58 A

(iii) When the coils A and B are connected in parallel, equivalent resistance in the circuit,
1/R_p = 1/24 + 1/24 = 2/24
or R_p = 12 Ω
Therefore, current through the parallel combination, i.e.,
I = V/R = 220/12 = 18.3 A

Question: Compare the power used in the 2 Ω resistor in each of the following circuits.
(i) a 6 V battery in series with 1 Ω and 2 Ω resistors, and
(ii) a 4 V battery in parallel with 12 Ω and 2 Ω resistors.

Answer:

(i) Since 2 Ω and 1 Ω resistors are in series therefore, equivalent resistance is
R_s = 2 + 1 = 3 Ω
Hence, current through the combinations
I = V/R = 6/3 = 2 A
Hence, power consumed by 2 Ω resistor
P_s = I²R = (2)² × 2 = 8 W

(ii) Since 12 Ω and 2 Ω are in parallel, therefore, potential across 2 Ω resistor is 4 V
Hence, power consumed by 2 Ω resistor is
P_p = V²/R = (4)²/2 = 8 W

Thus, in both cases the power consumed by the 2 Ω resistor is 8 W. Therefore, ratio of power consumed in the two cases is one.

Question: Two lamps, one rated 100 W at 220 V, and the other 60 W at 220 V, are connected in parallel to electric mains supply. What current is drawn from the line if the supply voltage is 220 V?

Answer: Given P₁ = 100 W, 220 V and
P₂ = 60 W, 220 V
In parallel combination of lamps, the total power consumed is given by
P = P₁+ P₂
Therefore, we have
P = 100 + 60 = 160 W
Since P = VI, therefore,
I = P/V = 160/220 = 0.727 A

Question: Which uses more energy, a 250 W TV set in 1 hr, or a 1200 W toaster in 10 minutes?

Answer:
Energy consumed by 250 W TV set in 1 h = 250 x 1 = 250 Wh.
Energy consumed by 1200 W toaster in 10 min = 1200 X 1/6 = 200 Wh.
∴ Energy consumed by TV set is more than the energy consumed by toaster in the given timings.

Question: Explain the following.
(1) Why is the tungsten used almost exclusively for filament of electric lamps?
(2) Why are the conductors of electric heating devices, such as bread-toasters and electric irons, made of an alloy rather than a pure metal?
(3) Why is the series arrangement not used for domestic circuits?
(4) How does the resistance of a wire vary with its area of cross-section?
(5) Why are copper and aluminum wires usually employed for electricity transmission?

Answer:

1. It has high melting point and emits light at a high temperature.
2. It has more resistivity and less temperature coefficient of resistance.
3. (i) All appliances do not get same potential in series arrangement.
   (ii) All appliances cannot be individually operated.
4. R ∝ =1 / Area of cross-section.
5. They are very good conductors of electricity.